We're living in the 21st century, and yet there's a dearth of cities on the Moon, gigantic space stations, asteroid mines, human expeditions to Mars and Jupiter, and all the other cool stuff that we'd expected to have by now. NASA and the other space agencies have totally failed to deliver this science fictional future for us, and I for one feel like I've been conned. In this article and its sequels I will explain my understanding of the reasons that we don't live in that world and the ways in which we still could. Like so many other things, it all comes down not to technology but to economics.
The fundamental currencies of space travel are Δv ("delta-vee") and reaction mass. To put a spacecraft onto a given trajectory requires imparting to it a certain amount of speed. Changing to another trajectory then requires another burn of the ship's engines to change the speed once more. The Δv of a mission is the (scalar) sum of all these changes in speed. For a given itinerary across the solar system, the Δv depends entirely on the laws of mechanics and is entirely independent of engineering details. Reaction mass, on the other hand, is very much an engineering detail: it's the amount of mass that the spacecraft can throw out to produce acceleration. It's conventional to think of reaction mass not in terms as an absolute mass but as a reaction mass fraction, which is the fraction of the ship's mass that is reaction mass: a spacecraft with a high mass fraction carries a lot of fuel for a given amount of payload.
The key thing about the Δv requirements for getting around the solar system is that there's a minimum amount required for any given trip. The trajectories that use the absolute minimum Δv are called Hohmann transfer orbits. The basic idea is that you start out in some circular orbit, burn your rockets to move onto an elliptical orbit ("injection"), and then burn them again at the destination to move back onto a circular orbit ("capture"). The elliptical orbit is carefully chosen so that it's tangent to both the source and target orbits. This in turn means that you have to plan your trip to coincide with certain launch windows during which these conditions are satisfied.
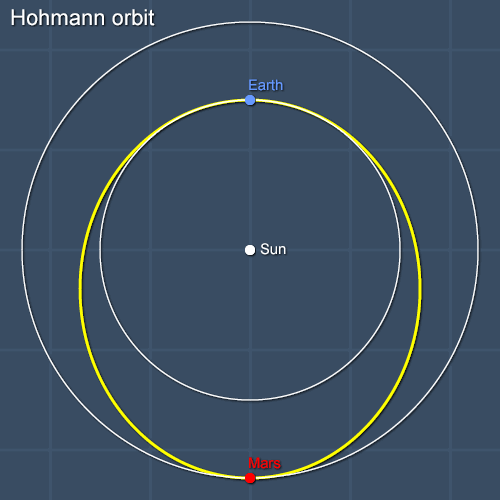
It's certainly possible to get to your destination faster if you're willing to use a higher Δv, but you can't get there unless you use the Hohmann orbit's minimum Δv, no matter how slowly you'd be willing to go. If you're lucky and you're going somewhere with some atmosphere, you can provide the Δv at the target by "aerocapture", which means using the drag of the atmosphere to slow you down relative to the target. Here are some figures for Δv requirements for some key trips around the solar system:
| Trip | Δv / km s-1 |
|---|---|
| Low Earth orbit to NEO | 5.0 |
| Low Earth orbit to Lunar surface | 6.1 |
| Low Earth orbit to Mars injection | 3.7 |
| Aerocapture at Mars | Free |
| NEO to Earth injection | 1.0 |
| Lunar surface to Earth injection | 3.0 |
| Mars surface to Earth injection | 7.8 |
| Aerocapture at Earth | Free |
From these Δv values, let's build three reference missions. Firstly, a typical near-Earth object reference mission that goes out from low Earth orbit (LEO) to a near-Earth asteroid (NEO, Near Earth Object), mines some material, and brings the mined material back to LEO. Secondly, a Lunar reference mission that goes from LEO to the Moon and returns mined material to LEO. Thirdly, a Mars reference mission that returns Martian material to LEO. Assuming that the aerocapture requirements are technologically feasible (which they might not be!), these missions have the following Δv requirements:
| Target | Δv / km s-1 |
|---|---|
| NEO | 6.0 |
| Luna | 9.1 |
| Mars | 11.5 |
The exchange rate between the Δv of a trip and the required reaction mass depends on the exhaust velocity of the ship's rocket system, and this in turn is set by the technology used in that rocket. For a given exhaust velocity, the required mass fraction varies exponentially with the required Δv, which is the first major problem with the industrialisation of space. In contrast, the Δv that can be achieved with a given mass fraction varies linearly with the exhaust velocity of the drive. Increasing the exhaust velocity of our rockets would thus help to ameliorate the difficulties of of moving things around the solar system. The traditional way to make space travel easier is by using better rockets to provide more Δv for a given amount of reaction mass
The exhaust velocity of a rocket depends on how hot you can make the gas that you expel. For chemical rockets, this is limited by how much energy burning the propellant releases. It's not possible to do much better than the Space Shuttle Main Engine, which has an exhaust velocity of around 4.5 km s-1. It's this limitation on chemical rocket performance that has spurred the development of nuclear thermal rockets, which heat a propellant by passing it through a nuclear reactor. This greatly improves performance:
| Type | ve / km s-1 |
|---|---|
| Chemical | 4.5 |
| Solid-core fission | 10.0 |
| Liquid-core fission | 13.2 |
| Gaseous-core fission | 15.7 |
(The nuclear thermal rocket figures are the approximate performance of reference designs with hydrogen propellant.)
Let's assume that we have a mature space mining infrastructure that we've already paid for. We have mines on Luna, Mars and a near-Earth asteroid and a freighter that's refuelled in LEO, flies out to the mine and brings the mined material back. Let's further assume that the mass of the cargo carried and of the reaction mass is much greater than that of the rockets and other systems. Given all this we can figure out how much fuel we need in LEO for each tonne brought back:
| Target | Fuel mass (chemical) | Fuel mass (nuclear) |
|---|---|---|
| NEO | 0.8t | 0.2t |
| Luna | 3.7t | 0.6t |
| Mars | 10.6t | 1.7t |
This all means that the cost of resources from space mining in a mature industry using this scheme depends on cost of fuel in LEO. The cost of such fuel brought up from the surface of the Earth is dominated by the launch vehicle costs. With current and near-future vehicles, this is around $10,000/kg or $10m/tonne. This means that we can figure out a minimum bound on the cost of material from space mines:
| Source | Price/tonne (chemical) | Price/tonne (nuclear) |
|---|---|---|
| NEO | $8m | $2m |
| Luna | $37m | $6m |
| Mars | $106m | $17m |
So what is worth all that money? For comparison, the current price of gold is $13m/tonne. This means that it might be economically viable to mine gold from near-Earth asteroids (and maybe from Luna or Mars) if we're willing to write off the entire cost of research and development of a nuclear rocket and mining systems, and the costs of deploying said systems. With just chemical rockets, we might be able to profitably mine gold from NEOs if we write off all development costs. It seems like space mining is a non-starter. To make it economically viable, we need to reduce the price of fuel in low Earth orbit. In the second part, "The Economics of Interface Transportation", I will examine the detailed economics of launching cargo from the surface of the Earth to low orbit and show that the prospects of the price per unit mass falling substantially are very low. To industrialise space fully we will need radically new approaches. I'll describe those in part three.
Update (23/8/2003): As well as having removed some assumptions on 23/5/2003, I've made some small changes for clarity (as suggested in the comments) and added a new diagram of a Hohmann transfer orbit.

|

|
Ooops... I was considering a solid-core fission thermal rocket - something along the lines of NERVA or Timberwind. |

|
Just checking... LEO is Low Earth Orbit? NEO is Near Earth Orbit? If that's right, are these asteroids: Nearby, Orbiting Earth? Orbiting [the sun] Near Earth? Orbiting Near Earth's Orbit? OR is NEO Near Earth Object? And would that object be: An Object Near Earth? An Object passing Near Earth? And if the object's not always near Earth, would it be worthwile setting up mining infrastructure on it? AND what's "injection", in this context? |

|
I've always missed something important, haven't I? "LEO" is "Low Earth Orbit" - the sort of orbit that things like the Shuttles and ISS operate in. "NEO" is "Near Earth Object", which means an asteroid or comet with an orbit around the Sun near that of the Earth. (There are also, of course, lots of asteroids in the main belt between the orbits of Earth and Mars, and possibly even more than that in the "Trojan" and "Greek" clusters at the stable Jupiter-Sun Lagrange points.) I think you'd have to plan your mining infrastructure deployments and target asteroids very carefully to make it worthwhile, because the easier it is to get to an asteroid the less frequent the launch windows. This probably means that asteroid mining is going to be a multi-decade operation. As I said, a Hohmann transfer requires two rocket burns - one at the source and one at the destination. In this context, "injection" is the first burn, that puts you on an elliptical orbit that takes you to the vicinity of your destination. If you don't make your second burn or aerocapture you will fall along the orbit to get back to the point in space from which you originated, which is unfortunate because the planet or asteroid that you left from won't be there then (it'll have moved on around its orbit). If you're prepared to use more fuel, you can get onto "free return" trajectories that will compensate for this and make your journey much safer. |

|
Delta-v is largely unrelated to the cost of space travel; the cost of space travel is the cost of keeping people alive in a hostile setting: those cost presently run about $3 million per person day. |

|
impossible! |

|
I was reading John McFee's "Curve of Binding Energy" and scarring the hell out of myself, again, as my first read was in 1974. Ted Tayor the protagonist,worked on Project Orion while at Atomics International. Could you comment on how that propusion concept could impact your calculations. |

|
Very good discussion of the physics and economics of space industry and colonization. A similar discussion can be found in Pournelle's A Step Farther Out where he goes over the Delta-Vee calculations. But much of the space industry theory depended on non-rocket resources, such as mass drivers on the Moon to access lunar materials...and mass drivers spilling slag off asteroids, to produce thrust and move them close to Earth. Plus the production of energy for Earth itself as a major product that would spur this development. GE analyzed asteroid mining early in the 1960s, and focused on things like gold, platinum, iridium, palladium and other expensive and rare goods. |

|
Nice Hohmann transfer graph. What program did you use to make it? |

|
Rick, This is all now a bit long in the tooth (though very clear), and I wonder if you might update it in light of LCROSS etc. I'm specifically interested in your calculation of cost per tonne moved in your reference missions, which are calculated from LEO, with the assumption that "The cost of such fuel brought up from the surface of the Earth is dominated by the launch vehicle costs". What costs do you get if it's lifted (a bit) from a lunar pole and then dropped down the Earth's gravity well to LEO? I'm guessing the economics all looks pretty different? |
 |
Yes, that would probably change things. It's much harder then to calculate minimum bounds for prices of missions but I suppose the costs would be dominated by the cost of extracting water on the Moon. I have no idea how to even estimate that, I'm afraid. By the way, I recommend Wikipedia's article on Delta-v budgets, which probably has better figures than I do, and a nice diagram too. |
Thanks for that Rich. You explain it well. It's good to have some figures for the Δv of the different options. What sort of nuclear engine are you considering in your chemical vs nuclear comparison?